
Ronak Gupta is pursuing a PhD in fluid mechanics at…
Equations, like art, are often abstract, elegant, beautiful, and why not. They are as much the product of creativity as art has ever been, and often transcend their mathematical boundaries and brim into popular culture.
It’s difficult to pinpoint the most famous equation of all time. Maybe it’s the one describing the mass-energy-momentum equivalence, or maybe it’s Fermat’s last theorem, famous for its exacting proof that took centuries to figure out, or maybe it’s Boltzmann’s formula, which allowed a mathematical way to make sense of entropy.
Whichever the winner is, not many are likely to have bet on the the Navier-Stokes equations. In fact, few scientists outside of fluid dynamics know about them, and even fewer outside science. What makes this ignorance curious is these equations have benefited most people one way or another.
In high school, most of us would have first learnt about the use of mathematics to describe reality through Isaac Newton’s laws of motion. The second law in particular relates the sum of forces acting on an object to its acceleration: F equals a multiplied by the object’s mass. To illustrate, your teacher will likely used the examples of a cuboidal block, a spherical ball or a pulley. Have you ever wondered why your teacher didn’t use the example of water or air instead?
It’s because Newton’s laws for fluids isn’t exactly F = m × a. Instead, it’s called the Navier-Stokes equations, which look like this:
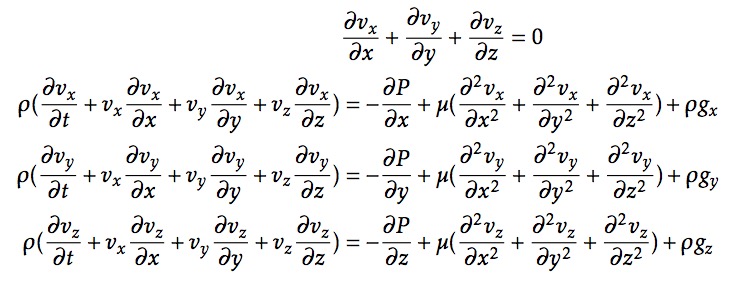
Here, the right hand side is essentially ‘F‘ and the left hand side is ‘m × a‘ for a fluid parcel. This is because Newton’s laws provide a relationship between force, mass and acceleration but for different systems, an application of the law results in an equation that doesn’t quite look like F = m × a but essentially is.
In the 19th century, a French civil engineer named Louis Navier combined the work of scientists before him – including Leonhard Euler, Joseph-Louis Lagrange and Augustin-Louis Cauchy – with lessons from his own experience working with hydraulics to lay the groundwork for modern fluid mechanics. Gabriel Stokes, a British mathematician, followed up with some independent observations of his own to complement the Frenchman’s findings. The result was a set of equations to calculate how weight, pressure and internal friction combine to change the velocity of fluid elements in space and time.
Our experience of this universe is in three spatial dimensions, so when we measure the velocity of water, we’re actually measuring the combination of its velocities in each of those dimensions, also called components. The Navier-Stokes equations provide one equation for each velocity component.
These three equations are then supplemented by a relation that enforces a condition called incompressibility. Water can’t be stretched or squashed. If you try to, it’s just going to flow instead. Incompressibility is the ability of a material to resist any change in volume, and it is keyed into the Navier-Stokes trio through an additional equation.
Also read: ‘If We Want New Revolutions, We Need New Abstract Concepts as Well’
Physicists and mathematicians have a deep knowledge of the physical relevance of the terms in these equations. We can also isolate the underlying mechanism in each case and explain why they are what they are using the first principles. However, the million-dollar question remains: are these equations guaranteed to work?
As surprising as it sounds, we don’t know.
Scientists have found that these equations predict how fluids will flow with excellent accuracy in a wide range of situations in carefully calibrated experiments. Clearly, the Navier-Stokes equations work, and we have empirical proof. But the mathematical proof that these equations will always work, without fail, remains out of reach.
Those working with pure mathematics in particular spend a considerable amount of time establishing the key properties of equations. In the Navier-Stokes case, they can be broadly divided into three.
1. Existence – Given a unique situation, will the equations always provide a solution?
2. Uniqueness – Will the solution be the one and only one?
3. Smoothness – Will the solution describe a fluid flow that is real?
The last bit is subtle but critical.
Imagine a stream of water is flowing down a channel. This stream can be divided into multiple parcels of water flowing along each other. If we can in theory measure the speed and direction of motion of all parcels individually, then its flow is called smooth. Real flow is supposed to be smooth, with directional and speed information available for all points in the flow. Such flow also has finite energy – energy that doesn’t arbitrarily accumulate at any point.
In other words, if we need to know that the Navier-Stokes equations actually work at all times, we simply need to know if their solutions have to do with finite numbers. But if we’re able to work out a condition in which the equations start spitting out infinities, then we’ll know that that’s the condition in which the equations don’t describe real flow.
In May 2000, the Clay Mathematics Institute in New Hampshire identified seven mathematical problems called the Millennium Prize Problems, and attached a $1 million bounty to the solution of each one. Proving that the Navier-Stokes equations fulfil their existence, uniqueness and smoothness conditions is one of them.
Also read: New Paper Hints at Solution for 160-Year-Old, Million-Dollar Math Problem
No one has claimed the prize; many have tried and failed. The equations have some wretched properties that render them near unmanageable. For example, they are coupled: the equation for one velocity component has terms that depend on other velocity components. They are nonlinear: some of the terms are proportional to the square of the velocity. They are also partial differential equations: they represent quantities that change with space as well as time at the same time.
Nonlinearity is often the biggest roadblock to solutions for equations that describe common natural phenomena. So an analytical solution to the Navier-Stokes equations has thus far been possible only under the most simplistic scenarios: when, for example, scientists make assumptions that reduce them to a linear form.
In the meantime, computers are helping them use the equations to solve real-world problems. Instead of obtaining a smooth solution that can give us the velocity of a parcel at any point in space and time, a computational solution yields a finite resolution version of it. This is like drawing a continuous line versus drawing while intermittently lifting the pencil. For almost any natural problem you can think of, the latter is good enough.
Years of tinkering have produced versions of the Navier-Stokes equations capable of describing the dynamics of different fluids and numerous phenomena. They have been used to model the ocean and the atmosphere on Earth, Jupiter’s Great Red Spot and pockmarks on Pluto. In some form or another, they have been invoked to study how bacteria swim and how airplanes fly. The equations have been found to be able to handle fluid flow at the molecular level with as much finesse as that at the galactic. It’s hard to oversell their power.
This is why it’s curious that they aren’t very well-known. It’s possible one reason is that Louis Navier and Gabriel Stokes never attained the sort of fame that 21st century physicists did. But their relative obscurity notwithstanding, the Navier-Stokes equations deserve a place in the panoply of great equations. They have occupied the minds of many of the brightest thinkers for centuries, and remain to this day objects of constant fascination, encompassing some of nature’s greatest secrets in only four lines. They are nothing less than art.
Ronak Gupta is doing a PhD in fluid mechanics at the University of British Columbia, Vancouver.

