
Interior side of a dome of a mosque in Isfahan, Iran. Photo: Nastaran Taghipour/Unsplash
- What should a mathematics course for humanities studies look like? To answer this, it would be instructive to consider two disparate views of mathematics.
- The first contends that mathematics is a tool that allows humankind to study the universe while the second is that mathematics is worthy of study for its own sake.
- The mathematics that students are typically exposed to in school is of the first type – the type that will also be ill-suited for humanities students.
- Instead, it will be better to have a course that reveals the beauty of number systems, sheds light on symmetry or showcases the creativity of geometric forms.
With the focus of the National Educational Policy on a broad-based education at the college level, every student of literature, the arts, philosophy, the social sciences – the humanities – will find herself taking at least one mathematics course in her college career.
What should such a course look like?
To answer this, it would be instructive to hold before us two disparate views of mathematics.
The first contends that mathematics is this wondrous tool that enables humankind to study the universe, be it in its impressively large length scale, the arena of galaxies and black holes, or in its equally impressive infinitesimal manifestation, where subatomic particles like quarks and leptons rule, or on a more human scale: how to build a stronger bridge, how to build a faster computer, how to study the spread of an epidemic.
The second view of mathematics is, alas, less widespread among the general public. It is that mathematics is first and foremost a thing of beauty. And it is its beauty that propels so many people to spend a lifetime studying mathematics for its own sake: the brilliance of the patterns contained within, the sheer elegance of the ideas involved in grasping its truths and, indeed, the awe felt when these majestic mathematical vistas are first espied, as if one is viewing divinity itself.
There is a reason why people rarely see this beauty. Most of their views of mathematics are formed from their exposure to the subject in school, and almost universally, mathematics in schools is taught as if it were just a tool that will eventually be of use in engineering or the sciences.
But school systems per se are not to blame. For one, it is difficult – although far from impossible – to communicate the beauty of mathematics at the early stages. For another, school systems are responsible for preparing students for a vast number of professions, many of which do need mathematics as a tool.
It is no wonder that a lot of high school mathematics consists only of manipulating symbols and practising algorithms (the “how to”) without going any deeper (into the “why”).
To students whose tastes and talents are geared more for the humanities, this sort of treatment of mathematics can, at least, appear bewildering. Unfortunately, it can also elicit more extreme reactions: for many students it has proven to be quite traumatic and has led to a lifelong fear and resentment of mathematics. This is a pity because mathematics is, at its core, an art.
There is a chance to begin to repair this gap in college, with a course on mathematics appreciation. It is possible to show glimpses of mathematics’ beauty without the long preparation required to grasp its advanced forms.
What would such a course consist of? It could reveal the beauty of number systems, shed light on symmetry and art, or showcase the imaginativeness of non-Euclidean geometry. These are just examples, there are many choices. The goal in each case should be to showcase the beauty of the subject, to illustrate mathematical reasoning, to explain how questions arise naturally in mathematics and how attempts to answer them reveal deeper and deeper phenomena.
Along the way, the course should reveal what every mathematician knows for sure: that mathematics is actually fun.
Consider the first suggestion. Mathematicians have plumbed the counting numbers (1, 2, 3, …) and have determined intricate structures in their midst. For example, the building blocks of this number theory are the prime numbers. There are infinitely many primes, and they show both patterns and randomness. And there are many unanswered questions about them: Are there infinitely many primes that differ by two, such as 5 and 7 or 17 and 19? Can every even number higher than four be written as a sum of two primes?
Perhaps the most tantalising is a conjecture called the Riemann hypothesis, whose proof (or disproof) is the holy grail of mathematics. It has been described by at least one mathematician as revealing the “music of the primes”.
Also read: What Is the Million-Dollar Riemann Hypothesis Problem?
A lovely beginning course can be fashioned out of this material by considering just the first steps that lead to these problems: for example, by proving formally that every number from 2 onwards can be expressed as a product of prime numbers in one and only one way, and considering the myriad properties of the counting numbers that flow from this.
Another suggestion: mathematicians have discovered abstract objects called groups that help make sense of symmetry. Consider a wallpaper pattern for example: its symmetry group is the collection of all ways to move the pattern around in space that leave the pattern unchanged to the eye.
The accompanying photo of a tile from Alhambra shows a pattern that can be rotated by 90º about each of the central stars – a demonstration of rotational symmetry. A wall made of these tiles would have a pattern that can be moved horizontally and vertically without changing the pattern – a demonstration of translational symmetry.
A course from the groups describing the symmetry of wallpaper designs could help teach the many patterns in Islamic art – as well as patterns that show up in nature such as in the arrangement of petals, the markings on wings or the shape of jellyfish.

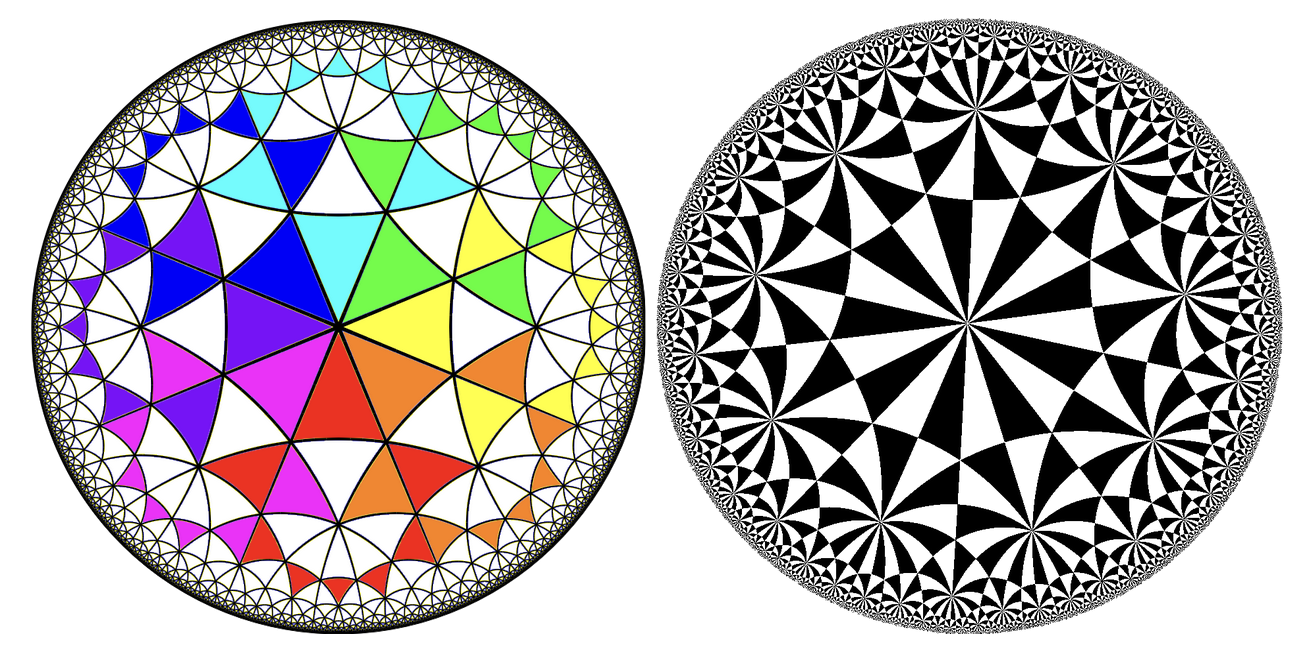
A third suggestion is also based on art. In Euclidean geometry, the sum of three angles of a triangle is 180º – but not so in non-Euclidean geometry.
There are two kinds of non-Euclidean geometry. In spherical geometry, the sum of the three angles of a triangle is greater than 180º, while in hyperbolic geometry, the same sum is less than 180º.
Hyperbolic geometry leads to fascinating mathematical art, as the accompanying pictures show. Here, the various curved triangles live in the hyperbolic world, and all have the same area in that world. Even though to our eyes they appear to be getting smaller and smaller, they are all congruent to each other in their world. The Dutch artist M.C. Escher used such hyperbolic geometry in several of his masterpieces.
There are indeed many other courses that can be created. The focus of any of them shouldn’t be on the same symbolic manipulation and algorithmic techniques that created unpleasant experiences for so many of us in school.
Instead, they should be treated as an opportunity to showcase the elegance of arguments, the depth of concepts, the value of constantly questioning hypotheses, the power of imagination as well as reasoning, and the far-reaching consequences of abstraction.
And along the way, they should reveal the fun that is mathematics.
Bharath Sethuraman is a professor of mathematics, Krea University.

